The GL(2,R) Action, the Veech Group, Delaunay Decomposition

Acting on surfaces by matrices.

from flatsurf import translation_surfaces

s = translation_surfaces.veech_double_n_gon(5)

s.plot()

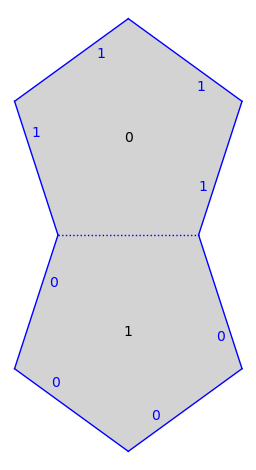
m = matrix([[2, 1], [1, 1]])

You can act on surfaces with the \(GL(2,R)\) action

ss = m * s
ss

Translation Surface in H_2(2) built from 2 pentagons

ss.plot()

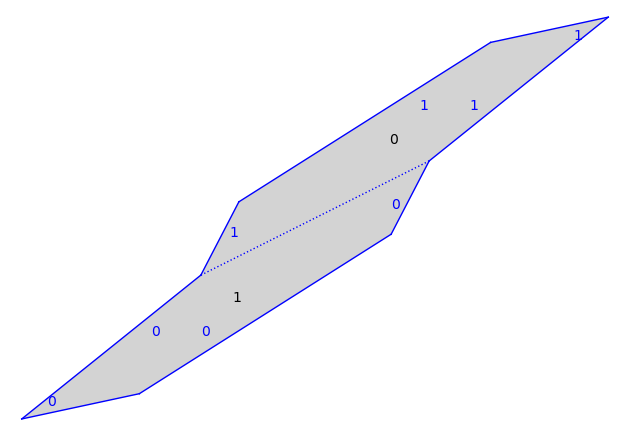
To “renormalize” you can improve the presentation using the Delaunay decomposition.

sss = ss.delaunay_decomposition()
sss

/home/runner/work/sage-flatsurf/sage-flatsurf/flatsurf/geometry/categories/similarity_surfaces.py:2891: UserWarning: delaunay_decomposition() has been deprecated and will be removed in a future version of sage-flatsurf; use delaunay_decompose().codomain() instead
  warnings.warn(

Delaunay cell decomposition of Translation Surface in H_2(2) built from 2 pentagons

sss.plot()

The Veech group

Set \(s\) to be the double pentagon again.

s = translation_surfaces.veech_double_n_gon(5)

The surface has a horizontal cylinder decomposition all of whose moduli are given as below

p = s.polygon(0)
modulus = (p.vertex(3)[1] - p.vertex(2)[1]) / (p.vertex(2)[0] - p.vertex(4)[0])
AA(modulus)

0.3632712640026804?

m = matrix(s.base_ring(), [[1, 1 / modulus], [0, 1]])
show(m)

\(\displaystyle \left(\begin{array}{rr} 1 & \frac{2}{5} a^{3} \\ 0 & 1 \end{array}\right)\)

show(matrix(AA, m))

\(\displaystyle \left(\begin{array}{rr} 1 & 2.752763840942347? \\ 0 & 1 \end{array}\right)\)

The following can be used to check that \(m\) is in the Veech group of \(s\).

s.canonicalize() == (m * s).canonicalize()

True

Infinite surfaces

Infinite surfaces support multiplication by matrices and computing the Delaunay decomposition. (Computation is done “lazily”)

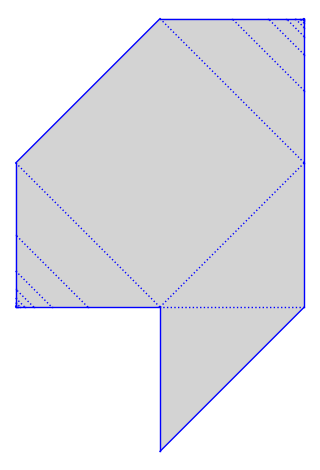
s = translation_surfaces.chamanara(1 / 2)

s.plot(edge_labels=False, polygon_labels=False)

ss = s.delaunay_decomposition()

gs = ss.graphical_surface(edge_labels=False, polygon_labels=False)
gs.make_all_visible(limit=20)

gs.plot()

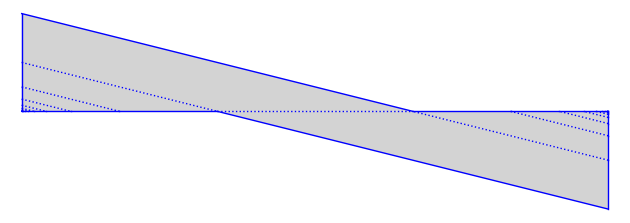
m = matrix([[2, 0], [0, 1 / 2]])

ms = m * s

gs = ms.graphical_surface(edge_labels=False, polygon_labels=False)
gs.make_all_visible(limit=20)
gs.plot()

mss = ms.delaunay_decomposition()

gs = mss.graphical_surface(edge_labels=False, polygon_labels=False)
gs.make_all_visible(limit=20)
gs.plot()

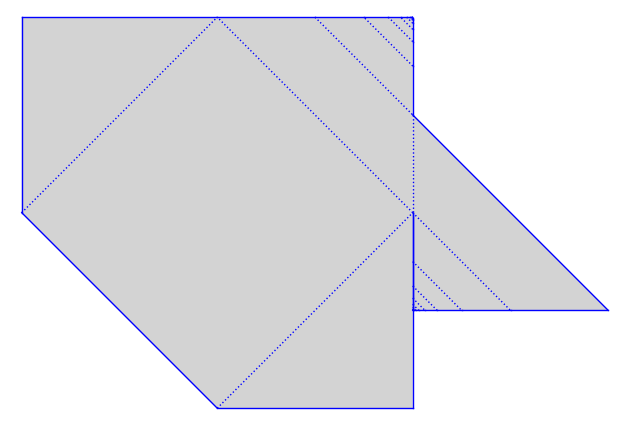
You can tell from the above picture that \(m\) is in the Veech group.